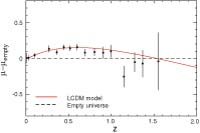
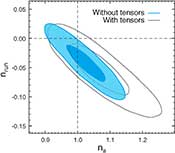
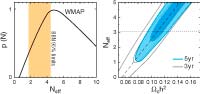
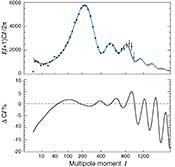
Likelihoods and Parameters
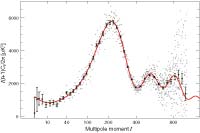
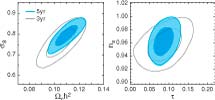
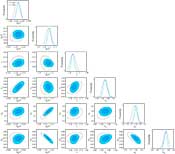
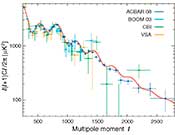
Five-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Likelihoods and Parameters from WMAP Data, J. Dunkley, et al., 2009ApJS..180..306D, reprint / preprint (1.2 MB) / bundled figures (312 KB) / individual figures / ADS / astro-ph
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||